Install Steam
login
|
language
简体中文 (Simplified Chinese)
繁體中文 (Traditional Chinese)
日本語 (Japanese)
한국어 (Korean)
ไทย (Thai)
Български (Bulgarian)
Čeština (Czech)
Dansk (Danish)
Deutsch (German)
Español - España (Spanish - Spain)
Español - Latinoamérica (Spanish - Latin America)
Ελληνικά (Greek)
Français (French)
Italiano (Italian)
Bahasa Indonesia (Indonesian)
Magyar (Hungarian)
Nederlands (Dutch)
Norsk (Norwegian)
Polski (Polish)
Português (Portuguese - Portugal)
Português - Brasil (Portuguese - Brazil)
Română (Romanian)
Русский (Russian)
Suomi (Finnish)
Svenska (Swedish)
Türkçe (Turkish)
Tiếng Việt (Vietnamese)
Українська (Ukrainian)
Report a translation problem





























































































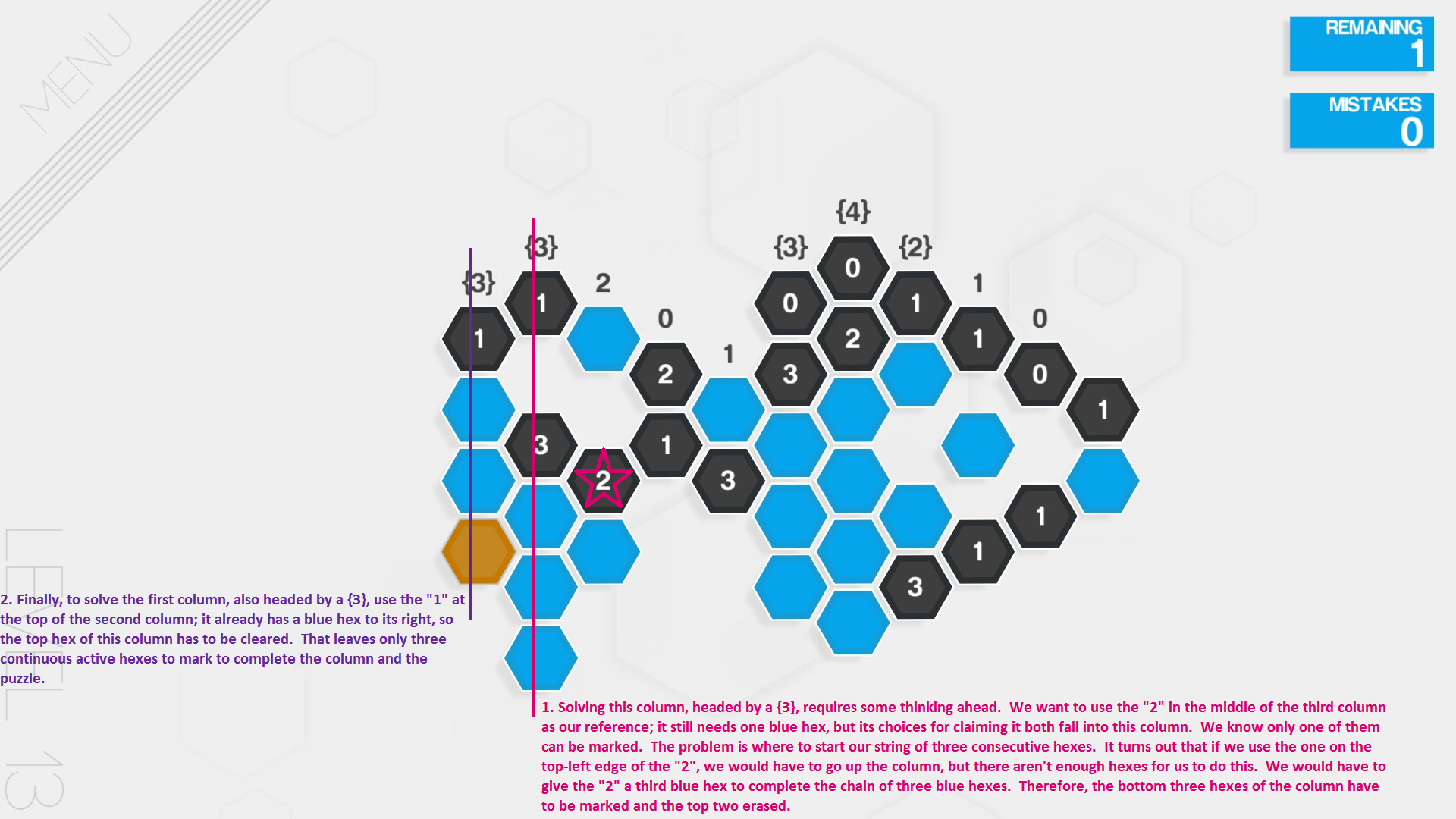























































































































































































I know that it has been a few years since I last posted on here. This has not been by choice, and I want to take a few minutes to talk more about what has happened to me and the new barriers I now face. I will be posting this to the comment threads to all of my Hexcells guides, so I apologize if you see it more than once. Also, due to Steam's extremely low character limit, I have to break this up into chunks. Please bear with me.
I'd like to thank you once again for the hard work you put in these guides and hope you have a great life, with better luck in the future!